An Introduction to Compound Interest

The concept of compounding can be complex and confusing to the average person without a background in finance. However, this is a concept that affects everyone who borrows or invests money. Whether you want to buy a car or a home, or you want to start a long-term savings plan for retirement, you need to understand how compound interest rates affect your payments. Breaking down this often confusing concept into easy to understand terms and examples will help you understand how to make the best financial decisions for your individual situation.

What is Compounding?

Source

Basically, compound interest means that the calculation of interest is done with the principal itself and the interest that has accumulated. This means that the loan (or investment) either decreases (or grows) at an increasing rate instead of a steady rate. This is why, when you look at your car, home, or student loan payment, the amount applied to principal vs the amount that goes to interest is always changing. The concept of compound interest is one of the most useful concepts to understand in the financial world. To understand how compound interest rates affect you personally, let’s look at borrowing and investing, both, separately with specific examples.

A Detailed Account of Borrowing Money at Compound Interest Rates

Regardless of the type of loan you want (home, auto, student loan), we calculate the compound interest through the same method. The interest that you will pay consists of the principal (of course) and the interest that accumulates over the life of the loan. We can illustrate how this works with a simple example.

If you borrow $100 at 10% interest compounded annually for three years, calculation of interest  is as follows:
$100 x 10% = $10.00 (year 1)
$110 x 10% = $11.00 (year 2)
$121 x 10% = $12.10 (year 3) = $33.10 total interest accrued after 3 years.

The difference between compound interest and simple interest is that calculation of simple interest starts with the principal:
$100 x 10% = $10 each year for $30 (simple) interest after 3 years

I

The calculation becomes harder when the period compounded is more often than just annually. Determining compound interest on loans: semi-annually, monthly, or even daily. The basic rule is that the higher the number of compounded periods, the greater the interest will be. For example, 5% interest compounded semiannually would be greater than 10% interest compounded annually ($34.00 vs $33.10)

Luckily, the manual calculation stays the same. However, punching the numbers for a loan when the compound interest accrues daily or even monthly gets ridiculous. The mathematical formula you can use: compound interest = principle x ((principal + interest) to the Nth power) minus the principal, where N = the number of compounded periods.

In our annual example, the formula would look like this: $100 ((100+.1) to the 3rd power) – $100 = $33.10. Again, doing the math is time-consuming. There are many websites that have a convenient compound interest calculator that is easy to use, such as the U.S. Securities and Exchange Commission website.

II

Now that you understand how to calculate compound interest, the final piece of the puzzle is understanding how making regular payments affects your balance. If you look at the breakdown of the payments you make, you see that part of the payment goes to interest and part of the payment goes to paying down the principal. If you track these payments over time, you see that the interest portion slowly goes down while the principal portion slowly increases. This makes sense when you understand that the principle of compound interest consists of both the principal and the interest. As the principal slowly gets paid down, the interest assessed is lower. While the payment amount stays the same, the amount applied to the principal slowly increases over time.

One of the benefits of compound interest loans is that you have the ability to pay the loan off faster and save money on the interest charges. When you pay extra on the principal, this brings down the cost of the loan. One example is a mortgage loan with no prepayment penalty. Just by paying a small additional amount on the principal each month can shave years off the life of the loan and save you thousands of dollars.

A Detailed Account of Investing Money at Compound Interest Rates

Source

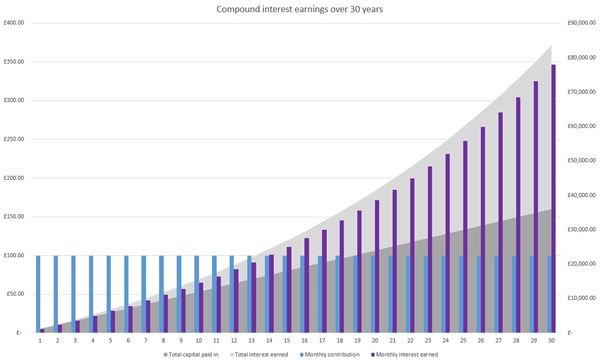
Just as borrowing money at compound interest rates affects how much your loan will cost you over time, investing money at compound interest rates will also have a huge impact on your long-term financial plans. When you are looking at a long-term savings plan for retirement, you want to make sure you are getting the maximum return on your money. Compound interest will add up faster over time because you are getting paid interest on your interest.

The calculations are the same. Looking at our simple example, investing $100 at 10% compounded annually will result in $133.10 after 3 years. Keep in mind that accruing periods make a difference. Different financial institutions use different periods. It’s standard for banks and savings institutions to accrue interest daily, although some offer a continuously compounding interest.

You must also see when the interest is actually put into your account. While the interest might be accruing daily, it might only be put into your account once a month. Interest only accrues on the amount that is actually in your account. This means that you won’t be earning “interest on interest”. At least, not until it does get deposited into your account.

Time Value of Money

The final concept that will help you understand compound interest is the time value of money. Have you ever wondered why the lump sum payout of a large sum of money (think lottery) is so much smaller than taking payments over time? The reason for this is that money you receive now is more valuable than money you would receive in the future. Money is more valuable now because you could invest it and over time it would grow to become a larger amount. Again, looking at our simple example proves this point. $100 now would be worth $133.10 in 3 years. So, $100 are more valuable now than $100 in 3 years.

This is a concept you need to understand when you have long-term savings goals. If you want enough money to maintain your lifestyle after retirement, you might set a goal of a certain amount in the future. Looking at the time value of money and compound interest rates allows you to set up a financial plan now to achieve your goals in the future.

Conclusion

Understanding the concept of compound interest will affect how you borrow and how you invest your hard-earned money. Once you understand how compounding works, you will be able to make the best financial decisions for your individual circumstances. While we have only provided the basics here, there will be another article on this subject. Feel free to leave comments, share your experiences, or ask questions. We welcome your input!